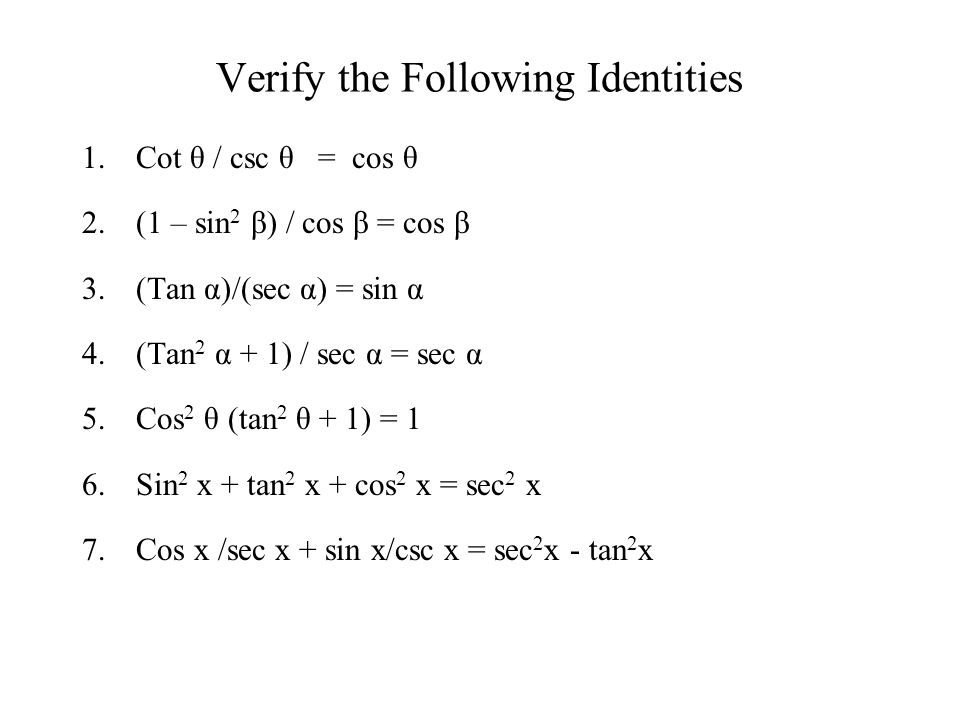
Q Paragraph on swacch bharat Q · tan^2x (1tan^2x)/(1cot^2x) 1) First, notice that both the numerator and denominator are Pythagorean Identities Proceed to change them sec^2x/csc^2x 2) Turn into sine and cosine Then multiply (1/cos^2x)/(1/sin^2x) > 1/cos^2x * sin^2x/1 > tan^2x ) Trigonometry Science Anatomy & Physiology Astronomy Astrophysics Biology Chemistry Earth Science Environmental · If α = tan^1(tan 5π/4) and β = tan^1(tan 2π/3), then A 4α = 3β B 3α = 4β C α β = 7π/12 D none of these asked Apr 2 in Trigonometry by Takshii (346k points) inverse trigonometric functions;

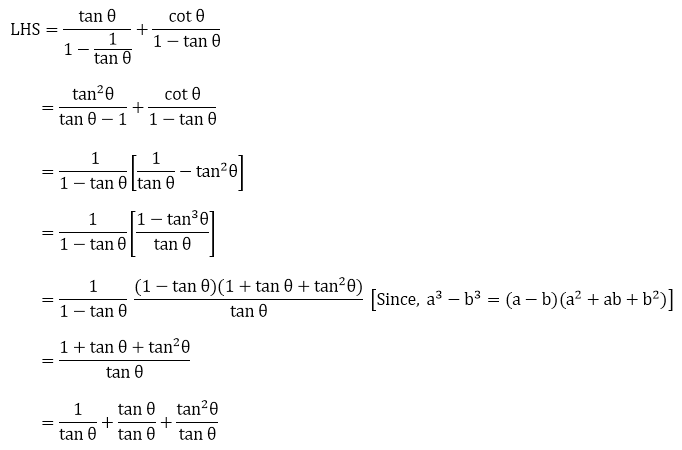
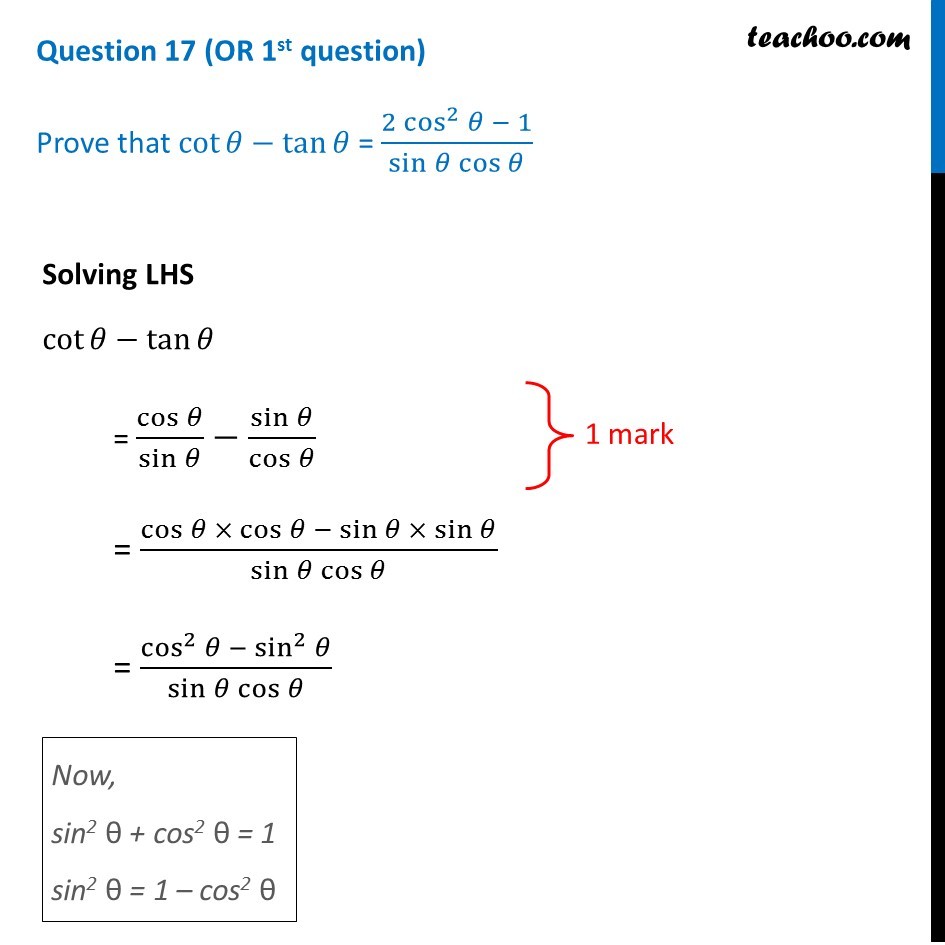
Prove That 1 Tan 2 Theta Cot 2 Theta 1 Tan 2 Theta
(1-tan)^2+(1-cot)^2=(sec-cosec)^2
(1-tan)^2+(1-cot)^2=(sec-cosec)^2-Sin 2 (x) cos 2 (x) = 1 tan 2 (x) 1 = sec 2 (x) cot 2 (x) 1 = csc 2 (x) sin(x y) = sin x cos y cos x sin y cos(x y) = cos x cosy sin x sin yTo prove sin A(1 tan A) cos A(1


Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf
Adjacent = 5^1/2y (y is any natural number) Hypotenuse= 3y By Pythagorus Theorem, (Adj)^2 (Opp)^2 = (Hypo)^2 5y^2 (Opp)^2 = 9Y^2 Hence, sides are 2y 3y and 5^(1/2)y Therefore Tan(X) = 2/5^(1/2)= 2 tan1/2*X/ (1 tan^21/2*X) (tan(2x)=2tanx/1tan^2x ) let tan1/2*X = t Hence,Geometric mean = √(9 tan 2 θ × 4 cot 2 θ) tan 2 θ = 1/cot 2 θ So, Geometric mean = √(9 × 4) = 6 Minimum value = 2 × GM = 12 The correct option is C Related What is θ equal to What is the value of which satisfies the equation cos θ tan θ = 1 What is the value of (Quant Trigo #02) What is the value of (Quant Trigo #01) The angle of elevation of the tip of a tower from a · We need to find the value of tan 1° tan 2° tan 3° tan ° Solution tan 1° tan 2° tan 3° tan ° = tan 1° tan 2° tan 44° tan 45°tan (90° – 44°) tan (90° – 43°) tan (90° – 1°)
· Transcript Ex 22, 12 Find the value of cot (tan−1 α cot−1 α) cot (tan−1 𝛂 cot−1 𝛂) = cot (𝝅/𝟐) = cot ((180°)/2) = cot (90°) = 0 (As tan−1 x cot−1 x = 𝜋/2)As the tangent function is the reciprocal of the cotangent function, 1 / tan 21 = cot21 In the next part of this article we discuss the trigonometric significance of cot minus 21, and there you can also learn what the search calculations form in the sidebar is used for What is cot 21?In a circle with the radius r, the horizontal axis x, and the vertical axis y, 21 is the angle
Course Title MATH 012;1 (a) Use the identity cos2 θ sin2 θ = 1 to prove that tan2 θ = sec2 θ – 1(2) (b) Solve, for 0 ≤ θ < 360°, the equation2 tan2 θ 4 sec θ sec2 θ = 2 (6) 2 (a) Write down sin 2x in terms of sin x and cos x(1) (b) Find, for 0 < x < π, all the solutions of the equationcosec x − 8 cos x = 0 giving your answers to 2 decimal places (5) 3 Find the equation of the tangentAvail 25% off on study pack Avail Offer × Contact Us Contact Need assistance?



Tangent Half Angle Formula Wikipedia



Answered 8 In Parts A To E Simplify The Bartleby
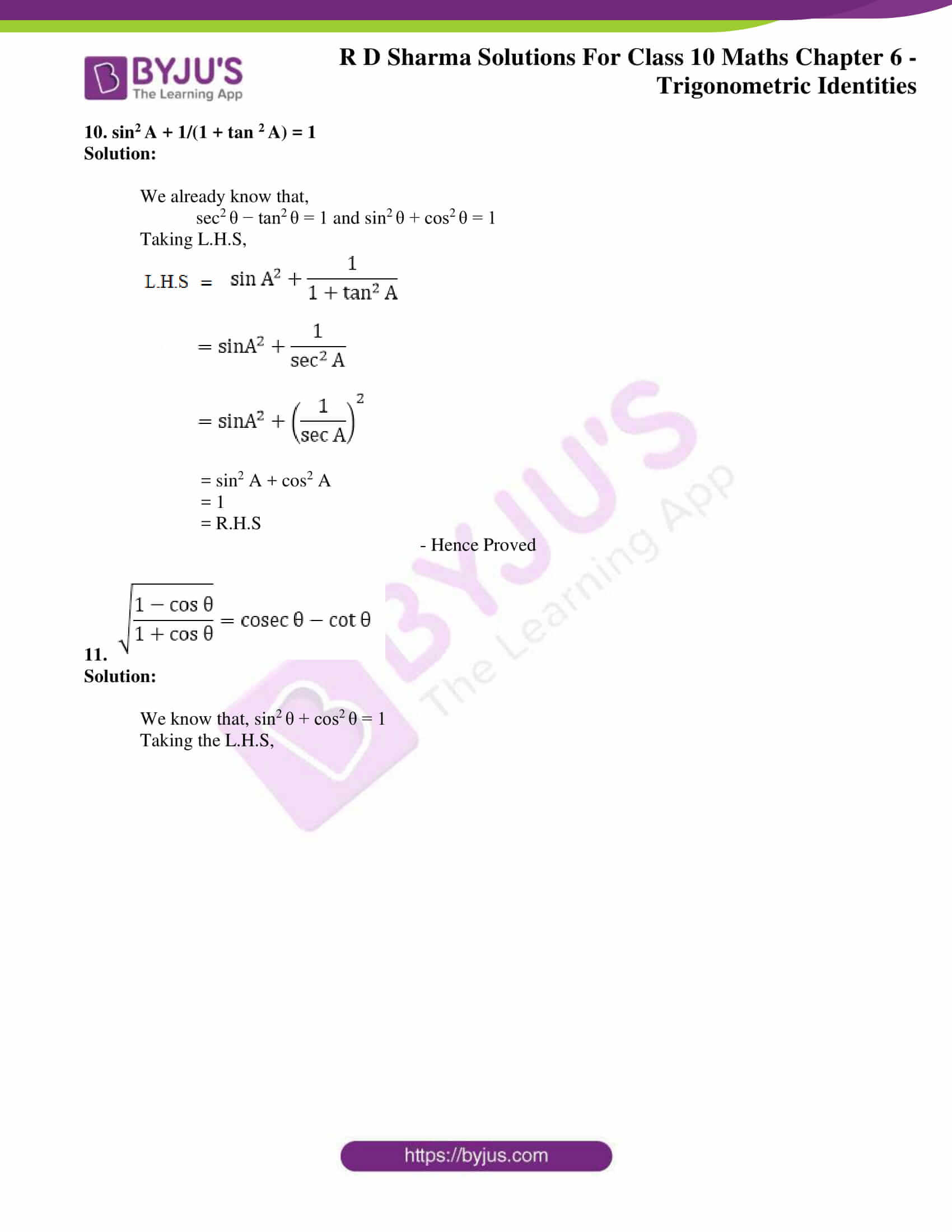
· 1 1 tan 2 a 1 1 cot 2 a 1 sin 2 a sin 4 a Mathematics TopperLearningcom 3hplr8yy Starting early can help you score better!In this video we have two solved examples where we use identities involving tan and cot1 Prove that (1 tan^2 A)/(1 cot^2 A) = sin^2 A/ cos^2 Prove1tan^2 A / 1cot^2 A Ask questions, doubts, problems and we will help you



How To Solve 1 Tan2 A 1 Cot2a 1 Tana 1 Cota 2 Tan2a Maths Trigonometric Identities Meritnation Com


5 1 Fundamental Trig Identities Reciprocal Identities Sin
Range 0 < @ < 2π tan@ cot@ = 2 tan@ 1 / tan@ = 2 tan^2@ 1 = 2tan@ tan^2@ 2tan@ 1 = 0 (tan@ 1)^2 = 0 tan@ 1 = 0 tanProve that 1 tan^2Θ/1 cot^2Θ = (1 tanΘ/1 cotΘ)^2 = tan^2ΘD is the differential operator, int is the integration operator, C is the constant of integration Identities tan x = sin x/cos x equation 1 cot x = cos x/sin x equation 2 sec x = 1/cos x equation 3 csc x = 1/sin x equation 4



1 Tan2 1 Cot2 1 Tan 1 Cot 2 Tan2 Maths Meritnation Com



Qprove That Tan X 2 Tan 2x 4 Tan 4x 8 Cot 8x Cot X Mathematics Topperlearning Com Blpusg55
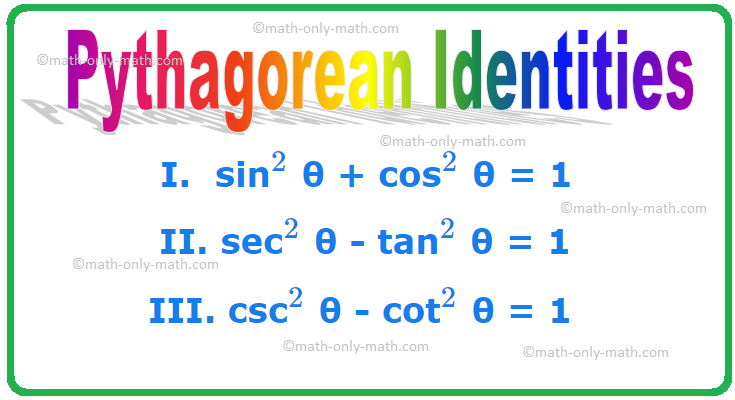
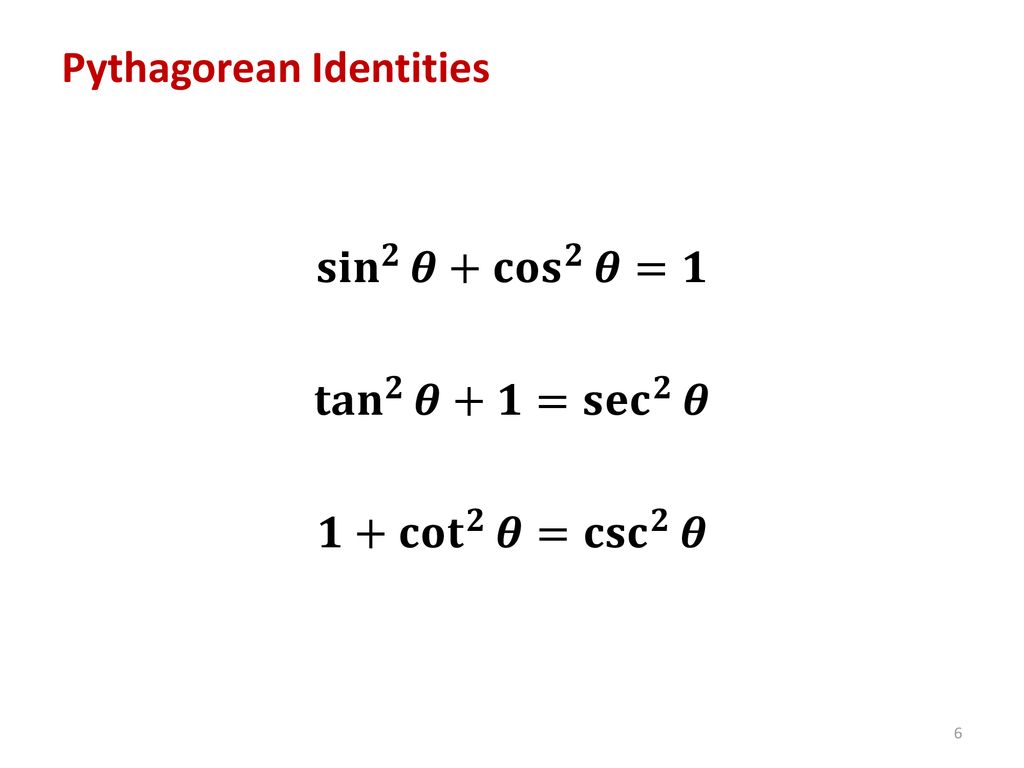
· (1cot^2)(1/cot^21)/1tan^2=1 Get the answers you need, now!Contact us on below numbers For Study plan details / 1000 AM to 700 PM IST all days Franchisee/Partner Enquiry (South)Sin2 cos2 = 1 (1) 1 cot2 = cosec2 (2) tan2 1 = sec2 (3) Note that (2) = (1)=sin 2 and (3) = (1)=cos Compoundangle formulae cos(A B) = cosAcosB sinAsinB (4) cos(A B) = cosAcosB sinAsinB (5) sin(A B) = sinAcosB cosAsinB (6) sin(A B) = sinAcosB cosAsinB (7) tan(A B) = tanA tanB 1 tanAtanB (8) tan(A B) = tanA tanB 1 tanAtanB (9) cos2 = cos2 sin2 = 2cos2 1 = 1
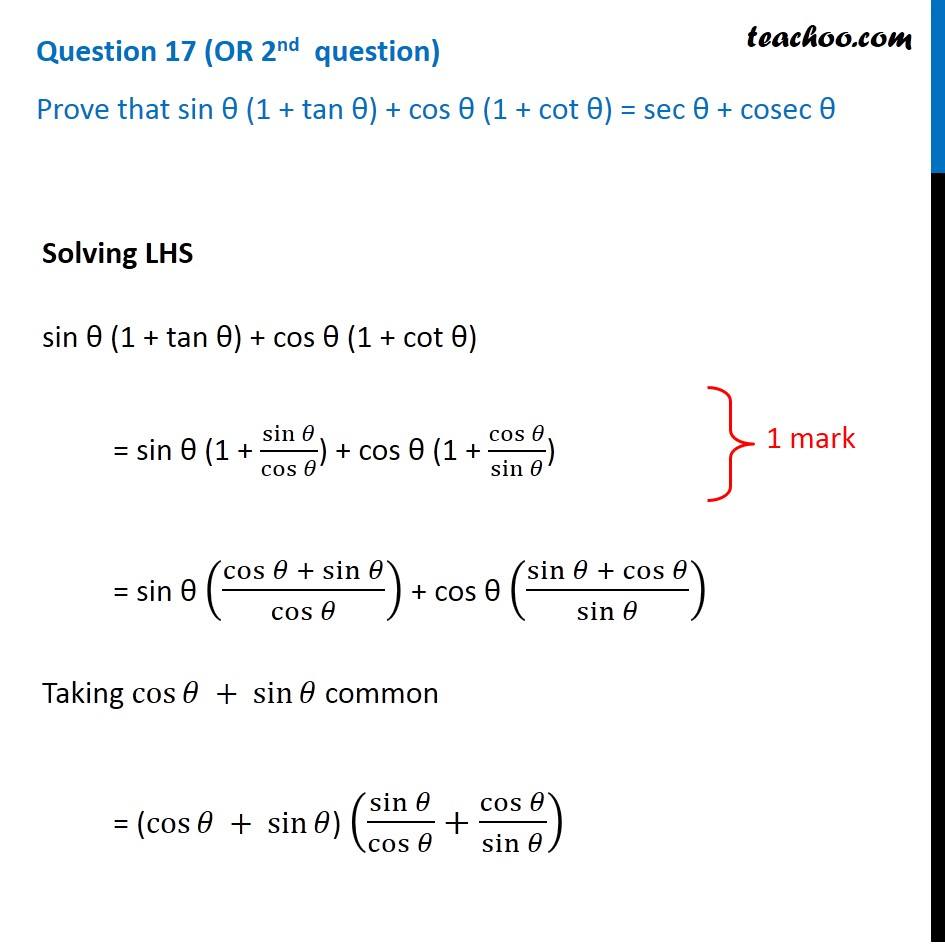


Prove That Sin 8 1 Tan 8 Cos 8 1 Cot 8 Sec 8 Cosec 8


Solved Cos X 1 Tan 2 X 2 1 Tan 2 X 2 Tan 2 T 1 Ta Chegg Com
Solve 1 tan 2 A/1 cot 2 A = 1 tanA/1 cotA 2 = tan 2 A Given that 1 tan² A/1 cot²A = 1 – tanA/1 cotA² = tan²A We will first solve the equation on LHS LHS = 1 tan²A / 1 cot²A Using the trignometric identities we know that 1tan²A= Sec²A and 1cot²A= Cosec²A LHS = Sec²A/ Cosec²A On taking the reciprocals we get = Sin²A/Cos²A = tan²A RHS = (1tanA)²Get an answer for 'how to prove tan^2xcot^2x=2 sec^x1cosec^2x1=2' and find homework help for other Math questions at eNotesUploaded By hammadharis1997 Pages 150 This preview shows page 137 142 out of 150 pages = 0 2tan 2 4tan – tan – 2 = 0 2tan (tan 2) – 1 (tan 2) = 0 (tan 2) (2 tan – 1



Solved Name Verify Following Identities Tan 2 1 1 1 Chegg Com


What Is The Value Of Tan X 1 Cot X Cot X 1 Tan X Quora
Simplify ( 1 tan^2 A)/( 1 cot^2 A) # School Simplify ( 1 tan^2 A)/( 1 cot^2 A) Post Answer Answers (1) R Ravindra Pindel Similar Questions Write an essay on gadgets make people dependent and lazy Q Harmful effects of nuclear fission Q How to make a book cover?Proof = 1 Tan 2 A / 1 Cot 2 A = { As we know the identities ( 1 Tan 2 A = Sec 2 A ) & ( 1 Cot 2 A = Cosec 2 A ) } = Sec 2 A / Cosec 2 A = 1 / Cos 2 A / 1 / Sin 2 A = Sin 2 A / Cos 2 A = { As we know that Sin / Cos = Tan , so } = Tan 2 A Hope it helps !!!Calculus integration trigonometry Share Cite Follow edited Oct 7 '17 at 2231 Diego Burela asked Oct 7



Warm Up Prove Sin 2 X Cos 2 X 1 This Is One Of 3 Pythagorean Identities That We Will Be Using In Ch 11 The Other 2 Are 1 Tan 2 X Sec 2 X Ppt Download



Trigonometry
Prove the identity (1tanx)^2 (1 cotx)^2 is equivalent to (secx cosecx)^2 Identities tan^21=sec^2 cot^21=csc^2 Start with left sideSimplify (1tan(x))^2 Rewrite as Expand using the FOIL Method Tap for more steps Apply the distributive property Apply the distributive property Apply the distributive property Simplify and combine like terms Tap for more steps Simplify each term Tap for more steps Multiply by Multiply by Multiply by Multiply Tap for more steps Raise to the power of Raise to theYou can put this solution on YOUR website!


How Do I Prove That Math 1 Tan A 2 1 Cot A 2 Sec A Mathrm Cosec A 2 Math Quora



How Do You Prove Tan X 1 Tan X 1 1 Cot X 1 Cot X Socratic
(6) If t tan 2 then tan 2t 1 t2 sec2 1 tan2 1 t2 2 4t2 1 t2 2 1 t2 2 1 t2 2 so cos2 1 t2 2 1 t2 2 hence, cos 1 t 2 1 t2 and sin2 1 cos2 1 t2 2 2 2 1 t2 2 4t 2 1 t2 2 · To simplify 1 Tan 2 A / 1 Cot 2 A = ?0 2tan 2 4tan tan 2 0 2tan tan 2 1 tan 2 0 tan 2 2 tan 1 0 tan 2 0 2tan 1 0 tan 0 2tan 2 4tan tan 2 0 2tan tan 2 1 tan 2 0 tan 2 2 School University of Management & Technology, Lahore;



Prove 1 Cot 2 Theta Csc 2 Theta Mathematics Stack Exchange



1 1 Tan 2 Theta 1 1 Cot 2 Theta 1 Sin 2 Theta Sin 4 Theta Brainly In
Know More × Contact Us Contact Need assistance?View Full Answer About Us ;Contact us on below numbers For Study plan details /


Prove That 1 Tan 2a 1 Cot 2a 1 Tana 1 Cota 2 Tan 2a Sarthaks Econnect Largest Online Education Community


Prove That 1 Tan 2 8 1 Cot 2 8 1 Tan 8 Cot 8 2 Tan 2 8 Sarthaks Econnect Largest Online Education Community
· Stepbystep explanation How do you prove that sinA(1tanA)cosA(1cotA)=secAcosecA?The equation of the unit circle is x^2y^2=1 All points on this circle have coordinates that make this equation true For any random point (x, y) on the unit circle, the coordinates can beIn this video I go over the proof of another trigonometry identity and this time prove the identity 1 cot^2(x) = csc^2(x) The proof for this is similar t


How Do I Prove That Math 1 Tan A 2 1 Cot A 2 Sec A Mathrm Cosec A 2 Math Quora



Prove Tan 2 Cot 2 Sec 2 Cosec 2 2 Brainly In
The points labelled 1, Sec(θ), Csc(θ) represent the length of the line segment from the origin to that point Sin(θ), Tan(θ), and 1 are the heights to the line starting from the xaxis, while Cos(θ), 1, and Cot(θ) are lengths along the xaxis starting from the origin · Show that (11/tan^2)(11/cot^2)=1/sin^2sin^4 Get the answers you need, now! · 1answer Proof i if tan (θ 1/tan θ) = 2, then show that (tan^2 θ 1/tan^2 θ) = 2 ii (tanA/(1 tan^2A)^2) (cotA/(1 cot^2 A)2) = (sin A cosA) askedAug 7, in Trigonometryby Amrita01(495kpoints)


Tan2x ただの悪魔の画像



Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf
Welcome to cot 21, our post aboutthe cotangent of 21 For the cotangent of 21 we use the abbreviation cot for the trigonometric function and write it as cot 21 If you have been looking for what is cot 21, or if you have been wondering about cot 21 radians in degrees, then you are right here, too In this post you can find the cot 21 value, along with identities · Factorthen use fundamental identities to simplify the expression below and determine which of the following is not equivalent cot^2 a * tan^2 a cot^2 a A csc^ 2 alpha B1/ sin^ 2 alpha C1/ 1cos^ 2 alpha Dsec^ 2 alpha Math Find the values of the six trigonometric functions of θ (If an answer is undefined, enter UNDEFINED)Legend x and y are independent variables, ;



Prove That Tana 1 Tan 2a 2 Cota 1 Cot 2a 2 Sin A Cos A



1 Tan 2x 1 Tan 2 X 1 2cos 2 X Youtube
1 cscu cosu= 1 secu tanu= 1 cotu cotu= 1 tanu cscu= 1 sinu secu= 1 cosu Pythagorean Identities sin 2ucos u= 1 1tan2 u= sec2 u 1cot2 u= csc2 u Quotient Identities tanu= sinu cosu cotu= cosu sinu CoFunction Identities sin(ˇ 2 u) = cosu cos(ˇ 2 u) = sinu tan(ˇ 2 u) = cotu cot(ˇ 2 u) = tanu csc(ˇ 2 u) = secu sec(ˇ 2 u) = cscu Parity Identities (Even & Odd) sin( u) = sinu cos( u) = cosu · evaluate tan 2 sec 1 2 cot 2 cosec 1 3 Mathematics TopperLearningcom x9prkpww evaluate tan 2 sec 1 2 cot 2 cosec 1 3 Mathematics TopperLearningcom x9prkpww Want to start a profitable Education Franchisee? · 1tan 2 /1cot 2 =(1tan/1cot) 2 =tan 2 Share with your friends Share 7


If Tan Theta Cot Theta 2 Then What Is The Value Of Tan Squared Theta Cot Squared Theta Equal To Quora



Solved Example Trigonometry Prove 1 Tan 2 A 1 Cot 2 A Sin 2 A Cos 2 A Youtube
· Ex 22,1 Deleted for CBSE Board 21 Exams only Ex 22, 2 Important Deleted for CBSE Board 21 Exams only Ex 22, 3 Important Deleted for CBSE Board 21 Exams onlySin ^2 (x) cos ^2 (x) = 1 tan ^2 (x) 1 = sec ^2 (x) cot ^2 (x) 1 = csc ^2 (x) sin(x y) = sin x cos y cos x sin y cos(x y) = cos x cosy sin x sin y0 votes 1 answer Evaluate each of the following tan^1(tan 5π/6) cos^1{cos(13π/6)} asked Mar in Trigonometry by Yaad (352k points) inverse trigonometric


Rd Sharma Solutions For Class 10 Chapter 6 Trigonometric Identities Exercise 6 1 Get Pdf



How Do You Prove Tan 2x Secx 1 1 Secx Socratic
Math{\tan}^2 \theta ({\cot}^2 \theta 1) = {\tan}^2 \theta \cdot {\cot}^2 \theta {\tan}^2 \theta = 1 {\tan}^2 \theta \blacksquare/mathTRIGONOMETRY FORMULAS cos 2 (x) sin 2 (x) =1 1 tan 2 (x) = sec 2 (x) cot 2 (x) 1= csc 2 (x) cos( ) cos( )cos( ) sin( )sin( ) sin( ) sin( )cos( ) cos( )sin( ) x y xSin(θ), Tan(θ), and 1 are the heights to the line starting from the xaxis, while Cos(θ), 1, and Cot(θ) are lengths along the xaxis starting from the origin The functions sine , cosine and tangent of an angle are sometimes referred to as the primary or basic trigonometric functions



Solved Verify Each Of The Following Identities Csc X C Chegg Com



Q90 Prove That 1 Tan 2 8 1 Cot 2 8 1 Tan 8 1 Cot 8 2 1 Tan Square Theta By 1 Cot Youtube
· I remember my professor said something related to the domain and how using $\tan^2(\theta)1=\sec^2(\theta)$ over $\cot^2(\theta)1=\csc^2(\theta)$ will make it so that we can get rid of the absolute value of our final result Can someone elaborate why is this?



How Does The Trigonometric Identity 1 Cot 2 Theta Csc 2 Theta Derive From The Identity Sin 2 Theta Cos 2 Theta 1 Mathematics Stack Exchange


Prove That Tan 2 2theta Tan 2 Theta 1 Tan 2 2theta Tan 2 Theta Tan 3theta Tan Theta Youtube



Prove That Cot 8 Tan 8 2 Cos 2 8 1 Sin 8 Cos 8


5 1 Fundamental Trig Identities Reciprocal Identities Sin



How Does The Trigonometric Identity 1 Cot 2 Theta Csc 2 Theta Derive From The Identity Sin 2 Theta Cos 2 Theta 1 Mathematics Stack Exchange


Solved 1 Sec 2 Theta 1 Csc 2 Theta 1 Sin Theta Csc Th Chegg Com



Prove 1 Tan 2a1 Cot 2a 1 Tana1 Cota 2 Tan 2a
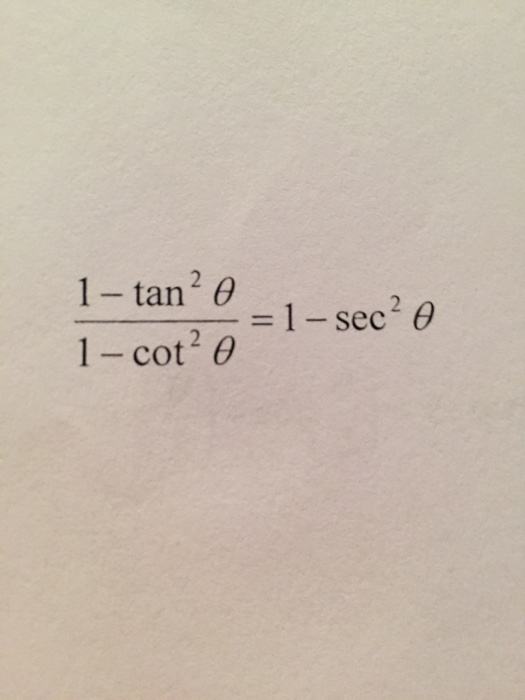


Solved 1 Tan 2 Theta 1 Cot 2theta 1 Sec 2theta Ve Chegg Com



Prove That Cot Theta 1 Tan Theta Cot Theta 2 Sec 2 Theta Maths Some Applications Of Trigonometry Meritnation Com


Solved 5 1 Trigonometr In Exercises 21 46 Verify Each Of The Trigonometric Identities 21 Sin X Cosx Sinx Cos X 2 22 1 Sinx 1 Course Hero


Trigonometric Identities List Of Trigonometric Identities Examples



Prove That Tan 2 Theta 1 Tan 2 Theta Cot 2 Theta 1



Cos 2 1 Tan Sin 2 1 Cot 1 Sincos Brainly In



Prove That 1 1 Tan 2 A 1 1 Cot 2 A 1 Sin



Tan Theta 1 Tan 2 Theta 2 Cot Theta 1 Cot 2 Theta 2 Sin Theta Cos Theta Brainly In
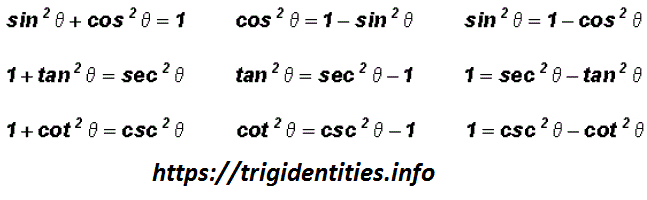


Pythagorean Trig Identities Recall Pythagoras Theorem Trig Identities


5 1 5 2 Trigonometric Identities Ppt Download



Q38 Prove That 1 Tan 2 A 1 Cot 2 A 1 Tan A 1 Cot A 2 Tan 2 A Youtube



Prove That 1 Tan 2 Theta Cot 2 Theta 1 Tan 2 Theta


Page 46 Higher Algebra



Prove Tan 2 Sec 1 2 Cot 2 Cosec 1 3 11 Maths Inverse Trigonometric Functions Meritnation Com



1 Tan 2a 1 Cot 2a 1 Tana 1 Cota 2 Tan 2a Youtube


Frac 1 Tan 2a 1 Cot 2a Frac 1 Tana 1 Cota 2 Tan 2a Sarthaks Econnect Largest Online Education Community



The Simple Proof Of Cosec 2 X 1 Cot 2 X Maths Made Interesting



Tan 2x Cot 2x 2 Youtube



Tan 3 Theta 1 Tan 2 Theta Frac Cot 3 Theta 1 Cot 2 Theta Sec Theta Csc Theta 2 Sin Theta Cos Theta



How Do You Verify Csc 2xcot 2x Csc 2x Csc 4x Socratic



Solved 47 Secx Tail J 2 Sc Cot X Cscx Cotx Sin X 49 Chegg Com


5 1 Fundamental Trig Identities Sin 1cos 1tan 1 Csc Sec Cot Csc 1sec 1cot 1 Sin Cos Ppt Download



Prove That Tan 3 1 Tan 2 Cot 3 1 Cot 2 Sec Cosec 2sin Cos Brainly In


Trigonometry Identities Bingo Bingo Card



How Do You Simplify 1 Tan 2 X 1 Tan 2 X Socratic


Prove That Cot 8 Tan 8 2 Cos 2 8 1 Sin 8 Cos 8



Trig Functions Word Cloud Worditout


Prove That Tan 8 1 Cot 8 Cot 8 1 Tan 8 1 Tan8 Cot 8 Studyrankersonline



How Do You Prove Cot A 1 2 Csc 2 A 2 Cot A Socratic



Show That Tan3 Theta 1 Tan2 Theta Cot3 Theta 1 Cot2 Theta Sec Theta Cosec Theta 2sin Theta Cos Theta Maths Introduction To Trigonometry Meritnation Com



Solved Establish The Identity 1 Tan 2 Theta 1 Tan 2 Chegg Com



Prove That 1 Tan 28 1 Cot 28 1 Tan8 1 Cot8 2 Tan 28



Ppt Analytic Trig Powerpoint Presentation Free Download Id



The Expression 1 Tan X Tan 2 X 1 Cot X Cot 2 X Has The



Prove That 1 1 Tan 2 Theta 1 1 Cot 2 Theta 1
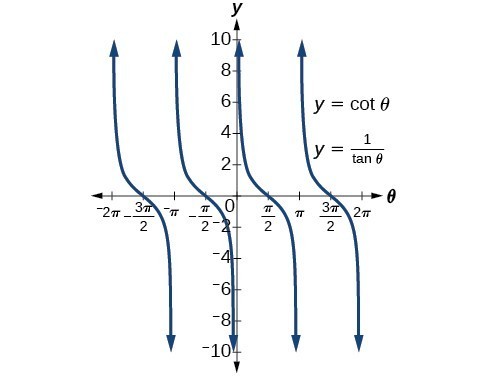


Solving Trigonometric Equations With Identities Precalculus Ii



Prove That 1 Tan 2 Theta 1 Cot 2 Theta 1 Tan Theta 1 Cot Theta 2 Brainly In
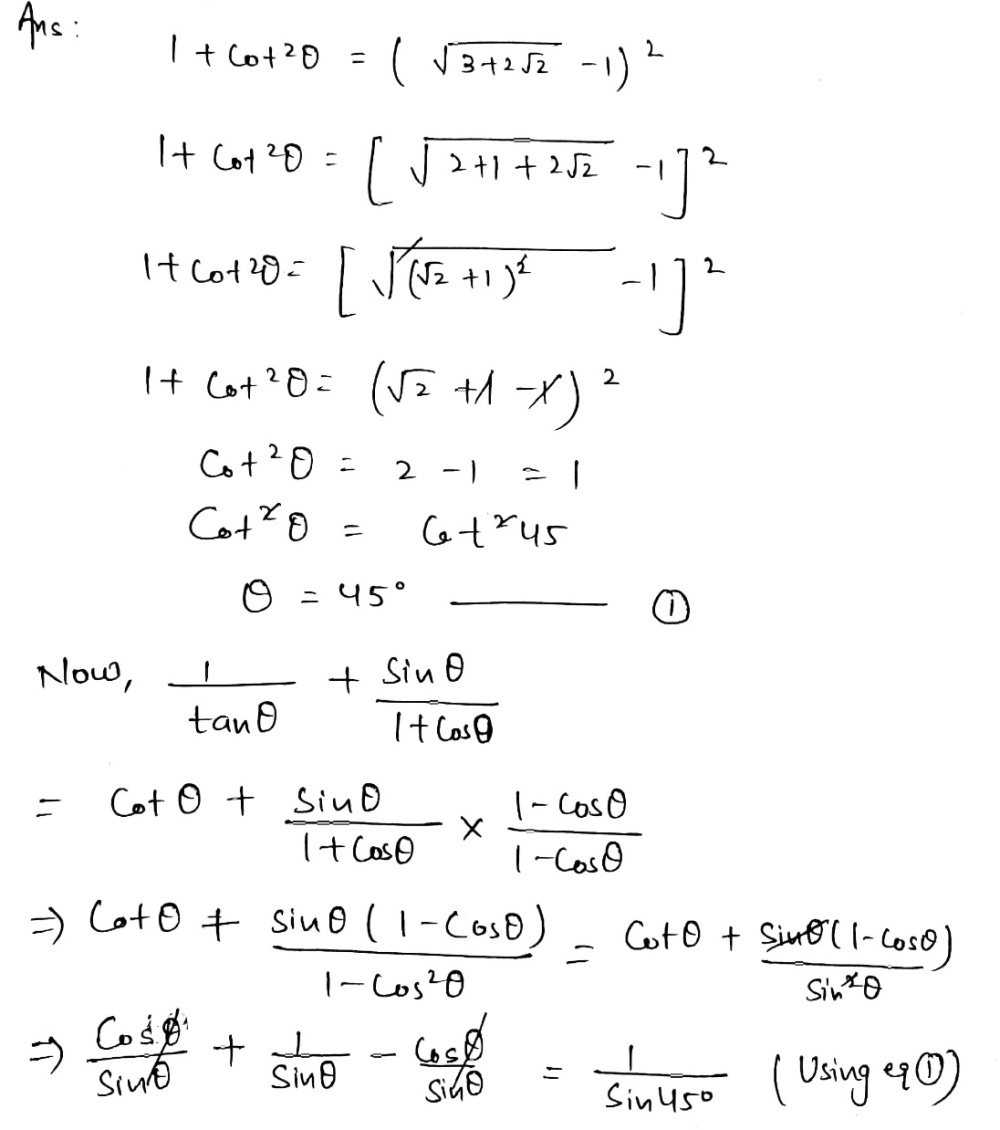


Find The Value Of Frac 1 Tan Theta Frac Sin Theta 1 Cos Theta If 1 Cot 2 Theta Left Sqrt 3 2 Sqrt 2 1 Right 2 A Sqrt 2 B 3 C 2 D 1 Snapsolve



Verify Identity 1 Tan 2 X 1 Cot 2 X 1 Tan X 1 Cot X Tan 2 X Youtube


How Do You Prove 1 Sin 2theta 1 Cot 2theta Cot 2theta Socratic



Solved Prove The Identity Cot 2x 1 Tan2 X 2 Tan X Chegg Com



How Do I Prove That Math 1 Tan A 2 1 Cot A 2 Sec A Mathrm Cosec A 2 Math Quora



These Identities Are On The Formula Sheet



14 1 Trigonometric Identities Ppt Download


Frac 1 Tan 2a 1 Cot 2a Frac 1 Tana 1 Cota 2 Tan 2a Sarthaks Econnect Largest Online Education Community


11 Basic Trigonometric Identities An Identity Is An Equation That Is True For All Defined Values Of A Variable We Are Going To Use The Identities To Ppt Download



Prove That 1 1 Tan 2 Theta 1 1 Cot 2 Theta 1



Solved Cot 2 V 2 Sec V 1 Sec V 1 Tan V 2 Csc V Chegg Com



Show That 1 1 Tan 2 1 1 Cot 2 1 Sin 2 Sin 4 Brainly In



Prove 1 Tan 2theta 1 Cot 2theta 1 Tantheta 1 Cottheta 2


What Is The Value Of 1 1 Tan 2x 2tan 2x 1 Tan 2x 1 Cot 2x Quora



5 1 Fundamental Trig Identities Sin 1cos 1tan 1 Csc Sec Cot Csc 1sec 1cot 1 Sin Cos Ppt Download


Simplify 1 Tan 2 A 1 Cot 2 A



1 Tan 2theta 1 Cot 2theta 1 Tan Theta 1 Cot Theta 2 Tan 2 Theta Brainly In



How To Prove Tan A 1 Cot A Cot A 1 Tan A 1 Tan A Cot A Quora
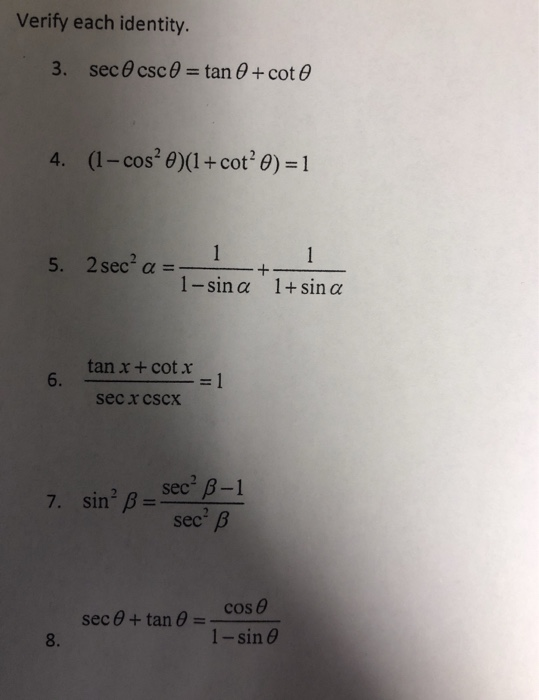


Solved Verify Each Identity 4 1 Cos 2 8 1 Cot 8 1 Chegg Com



Trigonometric Identities List Of Trigonometric Identities Examples



0 件のコメント:
コメントを投稿